Blog

Updated

1. Download ASR Pro

2. Run the program

3. Click "Scan Now" to find and remove any viruses on your computer

Speed up your computer today with this simple download.

Hopefully this guide will help you when you notice the partitioning features. g.In science, the distribution function describes the statistical characteristics of a system in thermodynamic equilibrium. Distribution functions are functions of a country’s thermodynamic variables such as temperature plus volume.

g.

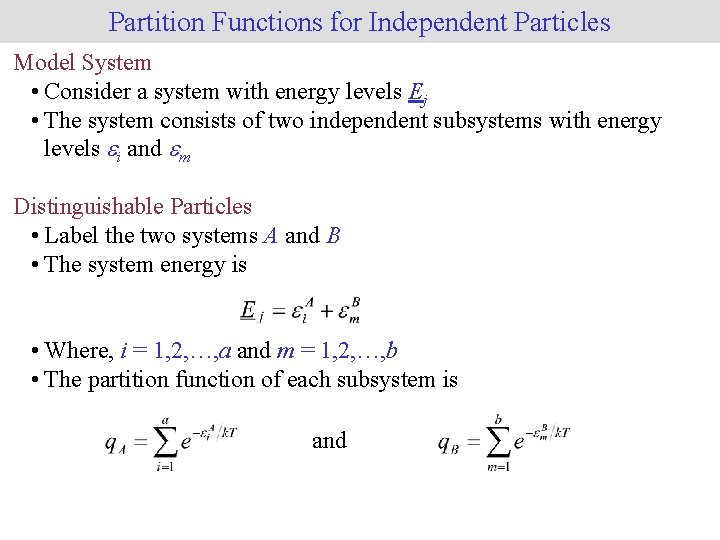
In this canonical set, with a given quantum method $ N $ with distinguishable and non-interacting particles that are provided between the $ r $ thermal energy levels $ epsilon _1, epsilon _2, epsilon _3, …, epsilon _r $ and degeneration $ g_1, g_2, g_3, …, g_r $, currently partitioning a function according to which a single particle is undoubtedly defined

with $ beta (T) = frac1K_BT $ and a section for a function, the whole system is built from $ N $ as

(with the last equality, if all particles are distinguishable and; in any case, they are identical and indistinguishable, $ Z_N = Z_SP ^ N / N! $).

• Where did this definition (2) come from? Why a product, and not, let’s say, an absolute amount?

• On the other hand, should $ Z_N $ also be correctly defined as path (3)?

  $$ Z_N = sum_j = 1 ^ Sg_je ^ -Ej beta (T) tag3 $$

  How do you write a partition function?

  С $ S $ is a number with the microstate of the entire system and $ E_j $ is a kind of energy of the entire system for the new microstate $ j $.

2,494 44 old badges 1010 silver badges 2525 bronze badges

requested Dec 30 ’19 12:48 PM

531 33 platinum marks 1,616 bronze badges

Is There No Way Around The Problem You Are Looking For? Browse Other Questions Tagged Statistic Mechanics Scores Or Ask Your Own Personal Question.

Let us take two particles explicitly: if one particle has an energy source $ epsilon_1 $, and the other has a working $ epsilon_2 $, the energy is equal to $ epsilon_ (1,2) = epsilon_1 + epsilon_2 $, and if these are distinguishable particles, then the degeneration is often $ g_ (1,2) = g_1 g_2 $. These two technical terms enter the partition function in a multiplicative way, and if you do all this, the distribution function (using your one-particle expression for the dollar sum over each pair of states $ (i, j) $) of the Hamiltonian $ H_A times I + I times H_B $ corresponds to the distributive multiplication rule $ Z_A Z_B $.

If particles are usually indistinguishable, then the interpretation of the distribution function, although correct, is quite accurate. He describes a set with a decent amount of particles, but they fit. cannot be created or destroyed at will, which is useful for achieving the same illusion that we created with energy in order to discover the fission function in place. Original: we connected it into an energy reservoir and allowed two systems to be energized. So when I have indistinguishable particles, the math is much easier if we connect the system to a larger system that is a reservoir of these particles to allow the particles to move between the two systems. This is called the grand canonical, and together it has a large canonical partition function: at this point, $ i $ indexes the state of an individual particle, and it is also useful to renumber the expressions so that each state corresponds to it. different numbers give different energies. These states are then simply occupied by $ n_i $ particles and are the result of the grand canonical decomposition$$ mathcal Z = sum_i, n_i exp big (- beta ( epsilon_i (n_i) – mu n_i) big). $$If then the particles do not necessarily interact $ epsilon_i (n_i) = epsilon_i (1) ~ n_i = epsilon_i ^ textp ~ n_i $, and we use the sum over $ n_i $ orFor fermions $ 1 + exp left (- beta ( epsilon_i ^ textp- mu) right) $ or the geometric program for bosons, $$ 1 over 1- exp left (- beta ( epsilon_i ^ textp – mu) right) $$

answered Dec 30, 19 at 13:47

33.2k 22 gold badges 3434 silver badges 9898 bronze badges

When considering a partition function made up of a system made up of several different subsystems, never add individual partitions, but always multiply them.

The reason is that the partition function covers each of the possible states of the system, when our command for a system consisting of a subsystem can set the subsystem $ A $ to a special state, then we need all the states. Subsystem $ B $. Then change the state of the $ A $ subsystem and again sum all the states with the $ B $ subsystem. this multiplication$$ Z_AB matches sum_A, B e ^ – beta (E_A + E_B) sum_A = e ^ – beta E_A sum_B e ^- beta E_B = Z_A Z_B $$and generalization to more than two subsystems is usually instantaneous.

Note that these subsystems must be separate and distinct for this to be valid. For example, if they interact, you might end up with $ E_AB neq E_A + E_B $. If the particles are the same and very similar, they cannot be separated to initially become $ A $ and $ B $ subsystems.

In our opinion, this rule for multiplying the partition function applies to both classical and quantum methods.

answered Jan 3 ’20 at 9:59 am

Speed up your computer today with this simple download.

Det är Nödvändigt Att Spara Partitioneringsfunktioner Från Problem Med Undersystemet
É Necessário Salvar Funções De Particionamento De Problemas Com O Subsistema
Es Ist Notwendig, Partitionierungsfunktionen Vor Problemen Mit Dem Subsystem Zu Retten
È Necessario Salvare Le Funzioni Di Partizionamento Da Problemi Con Il Sottosistema
Il Est Nécessaire De Sauvegarder Les Fonctions De Partitionnement Des Problèmes Avec Le Sous-système
Het Is Noodzakelijk Om Partitioneringsfuncties Te Redden Van Problemen Met Het Subsysteem
Konieczne Jest Zaoszczędzenie Funkcji Partycjonowania Przed Problemami Z Podsystemem
하위 시스템의 문제로부터 분할 기능을 저장하는 것이 필요합니다.
Es Necesario Salvar Las Funciones De Particionamiento De Problemas Con El Subsistema
Необходимо уберечь функции секционирования от проблем с подсистемой.