Table of Contents
Updated
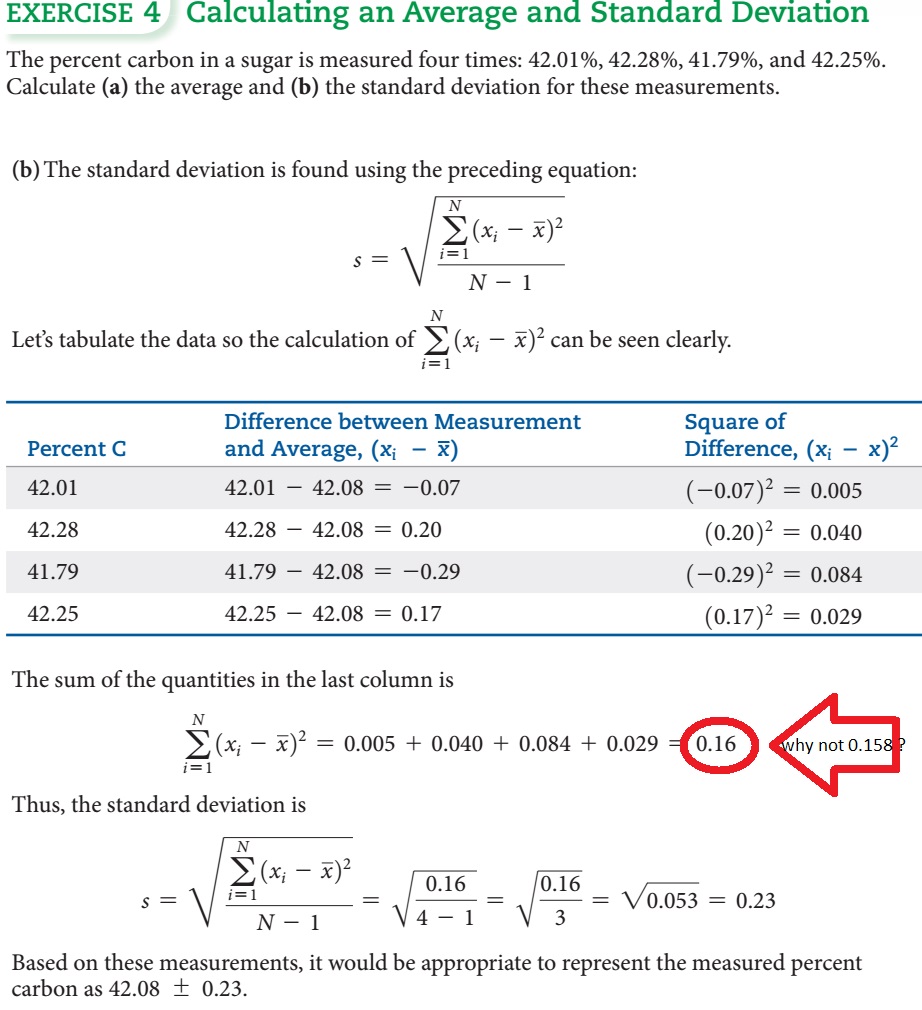
In this blog post, we will identify some possible causes that can lead to standard deviation rounding errors, and then point out possible fixes that you can try to resolve. g.The deviation of the rounding error quality, denoted by utes r, is then: Typically, the standard deviation to be rounded is a quarter of the difference between successive reporting values. To illustrate sheet thickness: due to rounding alone, the dimensional error should be at least 0.00025 ″.
g.Interpret
I answered this question by assuming that each experiment is performed 200 times. This experiment involves 25 people regardless of population (with replacement), and their average height is rounded to the nearest centimeter. The result is 200 product codes. You seem to be wondering, listen, what is the probability that a reasonable of these 200 numbers exceeds 176 cm.
This value requires that we deal with a small number of phenomena: the sample distribution of participation, rounding effects, and the end result of resampling. Other options are possible, but it looks like these problems will undoubtedly arise, so we hope that the following analysis will illustrate some useful and correct methods, even if a friend is actually assumed.th interpretation.
The sample distribution of the mean with 25 independent values (with substitution) has the same mean as the original distribution, and then 1/25 of its variance. At the same time, this is normal. In this case, the normal distribution (174.5; 6.9 / 5).
Rounding rotates the fixed distribution (in this case the normal (174.5, 6th in 9/5)) around the discrete distribution, since the possible final values are now 0, 1, …, 174, 175, 176.,. .., see The range of observation of one of these aspects $ y $ corresponds to the probability that true love is between $ y – 1/2 $ in addition to $ y 1/2 + $ and therefore
$$ Pr (Y) = Phi ( fracy 1/2 + – 174.56.9 / 5) – Phi ( fracy – 1/2 – 174.56.9 / 5). $$
where, as usual, $ Phi $ is the cumulative distribution function for finding the standard normal distribution.
Since the normal distributions are symmetrical, the rounding off should be compensated for by less than the mean. Balancing is perfect when the distribution mode is a huge integer, half thatthan indicated above. Thus, the mean of the unambiguous “discrete normal distribution” is 174.5.
How do you use standard error to round?
The standard errors should indeed be rounded to one decimal place, which is much more than the estimates for which they could well be calculated. Universe data can be queried without rounding. Sample survey data should be rounded. Zero measured in a universe control (that is, nothing at all) must always come from an array or number relative to zero.
Rounding can increase variance. As an approximation, people usually think that rounding is a random performing art, allowing a number to vary from an amount evenly divided between – $ -1 / $ 2 combined with + $ 1 / $ 2. The variance of this regular distribution is $ 1/12 $, from which we can estimate the variance of discrete normal daily use as follows

$$ sqrtsd ^ 2 + 1/2 = sqrt (6.9 / 5) ^ 2 + 1/12 corresponds to 1.40986 99703 63697 52354, $$
approximately. This approximation works when the rounding is very small compared to the standard deviation from the true distribution, which is the carrier here. In fact, exact calculations give a value of $ 1.40986 99703 63697 65285 $, which is 10 ^ -16 $ less than an approximation. This is more accurate than enough! “But it was worth checking out.
Updated
Are you tired of your computer running slow? Annoyed by frustrating error messages? ASR Pro is the solution for you! Our recommended tool will quickly diagnose and repair Windows issues while dramatically increasing system performance. So don't wait any longer, download ASR Pro today!

Now that people around the world know the distribution parameters associated with the rounded means of a sample of 25 people, namely the devilish174.5 and a standard deviation of 1.40986, we figure out how the expectation of 200 people used to be.The mean of these rounded averages is 174.5, and its standard deviation is $ 1.40986 / sqrt200 $ implies $ 0.099693. USA. This distribution should now be very close to normal, but it shouldn’t be quite that: after all, their value should be a multiple of 1/200 and equal to 0.005 cm. If you want you to be able to correct the continuity in the most important normal way, notice , which cannot be an average between 176 and 176.005 cm.In practice, however, this skill does not matter, since 5 1/2 inches is more than 15 standard revolutions above the average: it is almost impossible for an average of 200 rounded values could have exceeded 176.0025 or 176 .. The exact value is approximately
What is the rounding rule for standard deviation?
1. Having received a list of raw data, most peoplePeople should round off the mean and standard difference to one decimal place more than the exact data. If your data has no more decimal places, round to 0 decimal places. If your data has only one decimal place, round it to two decimal places.
, which is less than 10 ^ -53 $. Since the original population is “approximately” normal, we should not rely on such a low probability calculation. Suffice it to say that untangling is “practically nil”.
5. When you find the pattern, round up level n to the next level. the next larger number. Bordercolor = “# 888”
|
Information type |
Rounding rules |
| Another decimal place in addition to your data. | |
| One (E) error | Compare the number to the decimal places in the standard deviation. |
| Aspect ratio | Always up to three decimal places. |